Производная (asin(x))^(log(x))
Решение
Вы ввели
[src]
log(x) asin (x)
$$\operatorname{asin}^{\log{\left(x \right)}}{\left(x \right)}$$
d / log(x) \ --\asin (x)/ dx
$$\frac{d}{d x} \operatorname{asin}^{\log{\left(x \right)}}{\left(x \right)}$$
Подробное решение
-
Не могу найти шаги в поиске этой производной.
Но производная
Ответ:
Первая производная
[src]
log(x) /log(asin(x)) log(x) \
asin (x)*|------------ + -------------------|
| x ________ |
| / 2 |
\ \/ 1 - x *asin(x)/
$$\left(\frac{\log{\left(\operatorname{asin}{\left(x \right)} \right)}}{x} + \frac{\log{\left(x \right)}}{\sqrt{- x^{2} + 1} \operatorname{asin}{\left(x \right)}}\right) \operatorname{asin}^{\log{\left(x \right)}}{\left(x \right)}$$
Вторая производная
[src]
/ 2 \
log(x) |/log(asin(x)) log(x) \ log(asin(x)) log(x) 2 x*log(x) |
asin (x)*||------------ + -------------------| - ------------ + ------------------ + --------------------- + -------------------|
|| x ________ | 2 / 2\ 2 ________ 3/2 |
|| / 2 | x \-1 + x /*asin (x) / 2 / 2\ |
\\ \/ 1 - x *asin(x)/ x*\/ 1 - x *asin(x) \1 - x / *asin(x)/
$$\left(\left(\frac{\log{\left(\operatorname{asin}{\left(x \right)} \right)}}{x} + \frac{\log{\left(x \right)}}{\sqrt{- x^{2} + 1} \operatorname{asin}{\left(x \right)}}\right)^{2} - \frac{\log{\left(\operatorname{asin}{\left(x \right)} \right)}}{x^{2}} + \frac{x \log{\left(x \right)}}{\left(- x^{2} + 1\right)^{\frac{3}{2}} \operatorname{asin}{\left(x \right)}} + \frac{\log{\left(x \right)}}{\left(x^{2} - 1\right) \operatorname{asin}^{2}{\left(x \right)}} + \frac{2}{x \sqrt{- x^{2} + 1} \operatorname{asin}{\left(x \right)}}\right) \operatorname{asin}^{\log{\left(x \right)}}{\left(x \right)}$$
Третья производная
[src]
/ 3 2 \
log(x) |/log(asin(x)) log(x) \ 2*log(asin(x)) 3 /log(asin(x)) log(x) \ / log(asin(x)) log(x) 2 x*log(x) \ log(x) 3 2*log(x) 3 3*x*log(x) 3*x *log(x) |
asin (x)*||------------ + -------------------| + -------------- + ------------------- + 3*|------------ + -------------------|*|- ------------ + ------------------ + --------------------- + -------------------| + ------------------- - ---------------------- + -------------------- + -------------------- - ------------------- + -------------------|
|| x ________ | 3 3/2 | x ________ | | 2 / 2\ 2 ________ 3/2 | 3/2 ________ 3/2 / 2\ 2 2 5/2 |
|| / 2 | x / 2\ | / 2 | | x \-1 + x /*asin (x) / 2 / 2\ | / 2\ 2 / 2 / 2\ 3 x*\-1 + x /*asin (x) / 2\ 2 / 2\ |
\\ \/ 1 - x *asin(x)/ \1 - x / *asin(x) \ \/ 1 - x *asin(x)/ \ x*\/ 1 - x *asin(x) \1 - x / *asin(x)/ \1 - x / *asin(x) x *\/ 1 - x *asin(x) \1 - x / *asin (x) \-1 + x / *asin (x) \1 - x / *asin(x)/
$$\left(\left(\frac{\log{\left(\operatorname{asin}{\left(x \right)} \right)}}{x} + \frac{\log{\left(x \right)}}{\sqrt{- x^{2} + 1} \operatorname{asin}{\left(x \right)}}\right)^{3} + 3 \left(\frac{\log{\left(\operatorname{asin}{\left(x \right)} \right)}}{x} + \frac{\log{\left(x \right)}}{\sqrt{- x^{2} + 1} \operatorname{asin}{\left(x \right)}}\right) \left(- \frac{\log{\left(\operatorname{asin}{\left(x \right)} \right)}}{x^{2}} + \frac{x \log{\left(x \right)}}{\left(- x^{2} + 1\right)^{\frac{3}{2}} \operatorname{asin}{\left(x \right)}} + \frac{\log{\left(x \right)}}{\left(x^{2} - 1\right) \operatorname{asin}^{2}{\left(x \right)}} + \frac{2}{x \sqrt{- x^{2} + 1} \operatorname{asin}{\left(x \right)}}\right) - \frac{3 x \log{\left(x \right)}}{\left(x^{2} - 1\right)^{2} \operatorname{asin}^{2}{\left(x \right)}} + \frac{2 \log{\left(\operatorname{asin}{\left(x \right)} \right)}}{x^{3}} + \frac{3 x^{2} \log{\left(x \right)}}{\left(- x^{2} + 1\right)^{\frac{5}{2}} \operatorname{asin}{\left(x \right)}} + \frac{\log{\left(x \right)}}{\left(- x^{2} + 1\right)^{\frac{3}{2}} \operatorname{asin}{\left(x \right)}} + \frac{3}{\left(- x^{2} + 1\right)^{\frac{3}{2}} \operatorname{asin}{\left(x \right)}} + \frac{3}{x \left(x^{2} - 1\right) \operatorname{asin}^{2}{\left(x \right)}} - \frac{3}{x^{2} \sqrt{- x^{2} + 1} \operatorname{asin}{\left(x \right)}} + \frac{2 \log{\left(x \right)}}{\left(- x^{2} + 1\right)^{\frac{3}{2}} \operatorname{asin}^{3}{\left(x \right)}}\right) \operatorname{asin}^{\log{\left(x \right)}}{\left(x \right)}$$
График
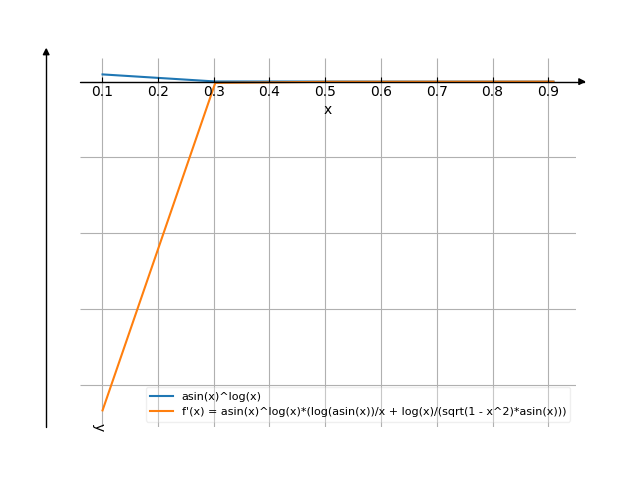

![Найти производную y' = f'(x) = (asin(x))^(log(x)) ((арксинус от (x)) в степени (логарифм от (x))) - функции. Найдём значение производной функции в точке. [Есть ОТВЕТ!] (asin(x))^(log(x))](/media/krcore-image-pods/176/hash/derivative/5/02/f808d7afa9c3d4c74e000101f90f1.png)
 Производная sqrt(1+x^2)*atan(x)
Производная sqrt(1+x^2)*atan(x)