Производная acos(x)^3*sin(x)
Решение
Вы ввели
[src]
3 acos (x)*sin(x)
$$\sin{\left(x \right)} \operatorname{acos}^{3}{\left(x \right)}$$
d / 3 \ --\acos (x)*sin(x)/ dx
$$\frac{d}{d x} \sin{\left(x \right)} \operatorname{acos}^{3}{\left(x \right)}$$
Первая производная
[src]
2
3 3*acos (x)*sin(x)
acos (x)*cos(x) - -----------------
________
/ 2
\/ 1 - x
$$\cos{\left(x \right)} \operatorname{acos}^{3}{\left(x \right)} - \frac{3 \sin{\left(x \right)} \operatorname{acos}^{2}{\left(x \right)}}{\sqrt{- x^{2} + 1}}$$
Вторая производная
[src]
/ 2 / 2 x*acos(x) \ 6*acos(x)*cos(x)\ -|acos (x)*sin(x) + 3*|------- + -----------|*sin(x) + ----------------|*acos(x) | | 2 3/2| ________ | | |-1 + x / 2\ | / 2 | \ \ \1 - x / / \/ 1 - x /
$$- \left(\sin{\left(x \right)} \operatorname{acos}^{2}{\left(x \right)} + 3 \left(\frac{x \operatorname{acos}{\left(x \right)}}{\left(- x^{2} + 1\right)^{\frac{3}{2}}} + \frac{2}{x^{2} - 1}\right) \sin{\left(x \right)} + \frac{6 \cos{\left(x \right)} \operatorname{acos}{\left(x \right)}}{\sqrt{- x^{2} + 1}}\right) \operatorname{acos}{\left(x \right)}$$
Третья производная
[src]
/ 2 2 2 \ 2
3 | 2 acos (x) 6*x*acos(x) 3*x *acos (x)| / 2 x*acos(x) \ 9*acos (x)*sin(x)
- acos (x)*cos(x) - 3*|----------- + ----------- - ----------- + -------------|*sin(x) - 9*|------- + -----------|*acos(x)*cos(x) + -----------------
| 3/2 3/2 2 5/2 | | 2 3/2| ________
|/ 2\ / 2\ / 2\ / 2\ | |-1 + x / 2\ | / 2
\\1 - x / \1 - x / \-1 + x / \1 - x / / \ \1 - x / / \/ 1 - x
$$- \cos{\left(x \right)} \operatorname{acos}^{3}{\left(x \right)} - 9 \left(\frac{x \operatorname{acos}{\left(x \right)}}{\left(- x^{2} + 1\right)^{\frac{3}{2}}} + \frac{2}{x^{2} - 1}\right) \cos{\left(x \right)} \operatorname{acos}{\left(x \right)} - 3 \cdot \left(- \frac{6 x \operatorname{acos}{\left(x \right)}}{\left(x^{2} - 1\right)^{2}} + \frac{3 x^{2} \operatorname{acos}^{2}{\left(x \right)}}{\left(- x^{2} + 1\right)^{\frac{5}{2}}} + \frac{\operatorname{acos}^{2}{\left(x \right)}}{\left(- x^{2} + 1\right)^{\frac{3}{2}}} + \frac{2}{\left(- x^{2} + 1\right)^{\frac{3}{2}}}\right) \sin{\left(x \right)} + \frac{9 \sin{\left(x \right)} \operatorname{acos}^{2}{\left(x \right)}}{\sqrt{- x^{2} + 1}}$$
График
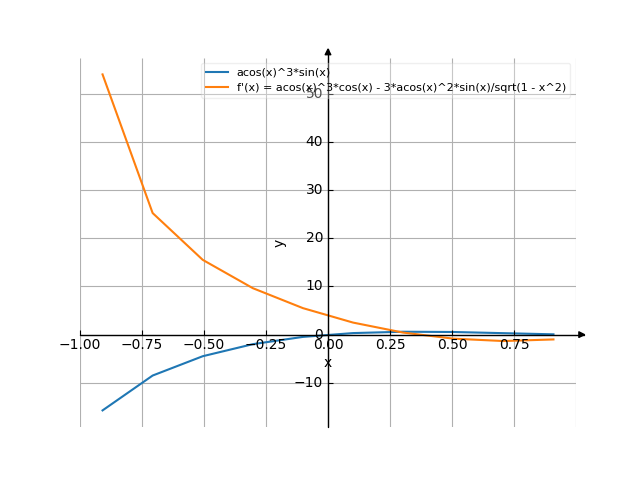

![Найти производную y' = f'(x) = acos(x)³*sin(x) (арккосинус от (x) в кубе умножить на синус от (x)) - функции. Найдём значение производной функции в точке. [Есть ОТВЕТ!] acos(x)^3*sin(x)](/media/krcore-image-pods/176/hash/derivative/d/d1/14ff41a7a646134066e27b90c4c23.png)