Производная atan(3*tan(x))/3
Решение
Вы ввели
[src]
atan(3*tan(x))
--------------
3
$$\frac{\operatorname{atan}{\left(3 \tan{\left(x \right)} \right)}}{3}$$
d /atan(3*tan(x))\ --|--------------| dx\ 3 /
$$\frac{d}{d x} \frac{\operatorname{atan}{\left(3 \tan{\left(x \right)} \right)}}{3}$$
Первая производная
[src]
2 3 + 3*tan (x) ----------------- / 2 \ 3*\1 + 9*tan (x)/
$$\frac{3 \tan^{2}{\left(x \right)} + 3}{3 \cdot \left(9 \tan^{2}{\left(x \right)} + 1\right)}$$
Вторая производная
[src]
/ / 2 \\
/ 2 \ | 9*\1 + tan (x)/|
-2*\1 + tan (x)/*|-1 + ---------------|*tan(x)
| 2 |
\ 1 + 9*tan (x) /
----------------------------------------------
2
1 + 9*tan (x)
$$- \frac{2 \cdot \left(\frac{9 \left(\tan^{2}{\left(x \right)} + 1\right)}{9 \tan^{2}{\left(x \right)} + 1} - 1\right) \left(\tan^{2}{\left(x \right)} + 1\right) \tan{\left(x \right)}}{9 \tan^{2}{\left(x \right)} + 1}$$
Третья производная
[src]
/ 2 2 \
| / 2 \ 2 / 2 \ / 2 \ 2 |
/ 2 \ | 2 9*\1 + tan (x)/ 54*tan (x)*\1 + tan (x)/ 324*\1 + tan (x)/ *tan (x)|
2*\1 + tan (x)/*|1 + 3*tan (x) - ---------------- - ------------------------ + --------------------------|
| 2 2 2 |
| 1 + 9*tan (x) 1 + 9*tan (x) / 2 \ |
\ \1 + 9*tan (x)/ /
----------------------------------------------------------------------------------------------------------
2
1 + 9*tan (x)
$$\frac{2 \left(\tan^{2}{\left(x \right)} + 1\right) \left(\frac{324 \left(\tan^{2}{\left(x \right)} + 1\right)^{2} \tan^{2}{\left(x \right)}}{\left(9 \tan^{2}{\left(x \right)} + 1\right)^{2}} - \frac{54 \left(\tan^{2}{\left(x \right)} + 1\right) \tan^{2}{\left(x \right)}}{9 \tan^{2}{\left(x \right)} + 1} + 3 \tan^{2}{\left(x \right)} - \frac{9 \left(\tan^{2}{\left(x \right)} + 1\right)^{2}}{9 \tan^{2}{\left(x \right)} + 1} + 1\right)}{9 \tan^{2}{\left(x \right)} + 1}$$
График
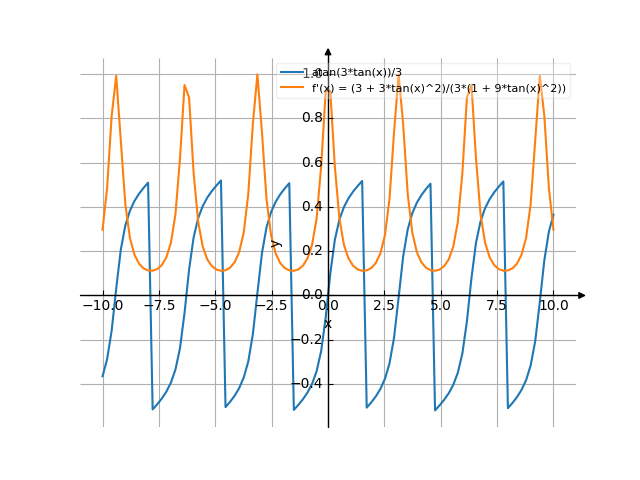

![Найти производную y' = f'(x) = atan(3*tan(x))/3 (арктангенс от (3 умножить на тангенс от (x)) делить на 3) - функции. Найдём значение производной функции в точке. [Есть ОТВЕТ!] atan(3*tan(x))/3](/media/krcore-image-pods/176/hash/derivative/e/c3/2e8fd64b7805e6ef6a82bb7a30db8.png)
 Производная log((5*x-3)/(2*x+7))
Производная log((5*x-3)/(2*x+7))
 Производная (3/x^2)+x^14
Производная (3/x^2)+x^14