Производная log(atanh((x)^(1/2)))
Решение
Вы ввели
[src]
/ / ___\\ log\atanh\\/ x //
$$\log{\left(\operatorname{atanh}{\left(\sqrt{x} \right)} \right)}$$
d / / / ___\\\ --\log\atanh\\/ x /// dx
$$\frac{d}{d x} \log{\left(\operatorname{atanh}{\left(\sqrt{x} \right)} \right)}$$
Первая производная
[src]
1
----------------------------
___ / ___\
2*\/ x *(1 - x)*atanh\\/ x /
$$\frac{1}{2 \sqrt{x} \left(- x + 1\right) \operatorname{atanh}{\left(\sqrt{x} \right)}}$$
Вторая производная
[src]
1 2 1
---- + -------------- - -----------------------
3/2 ___ / ___\
x \/ x *(-1 + x) x*(-1 + x)*atanh\\/ x /
-----------------------------------------------
/ ___\
4*(-1 + x)*atanh\\/ x /
$$\frac{\frac{2}{\sqrt{x} \left(x - 1\right)} - \frac{1}{x \left(x - 1\right) \operatorname{atanh}{\left(\sqrt{x} \right)}} + \frac{1}{x^{\frac{3}{2}}}}{4 \left(x - 1\right) \operatorname{atanh}{\left(\sqrt{x} \right)}}$$
Третья производная
[src]
3 1 1 1 3 3
- ------ - --------------- - --------------- - ------------------------------ + -------------------------- + --------------------------
5/2 ___ 2 3/2 3/2 2 2/ ___\ 2 / ___\ 2 / ___\
8*x \/ x *(-1 + x) 2*x *(-1 + x) 4*x *(-1 + x) *atanh \\/ x / 4*x*(-1 + x) *atanh\\/ x / 8*x *(-1 + x)*atanh\\/ x /
---------------------------------------------------------------------------------------------------------------------------------------
/ ___\
(-1 + x)*atanh\\/ x /
$$\frac{- \frac{1}{\sqrt{x} \left(x - 1\right)^{2}} + \frac{3}{4 x \left(x - 1\right)^{2} \operatorname{atanh}{\left(\sqrt{x} \right)}} + \frac{3}{8 x^{2} \left(x - 1\right) \operatorname{atanh}{\left(\sqrt{x} \right)}} - \frac{1}{2 x^{\frac{3}{2}} \left(x - 1\right)} - \frac{3}{8 x^{\frac{5}{2}}} - \frac{1}{4 x^{\frac{3}{2}} \left(x - 1\right)^{2} \operatorname{atanh}^{2}{\left(\sqrt{x} \right)}}}{\left(x - 1\right) \operatorname{atanh}{\left(\sqrt{x} \right)}}$$
График
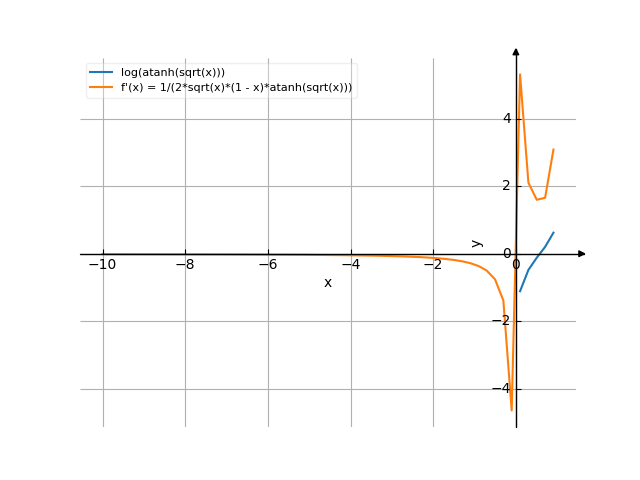

![Найти производную y' = f'(x) = log(atanh((x)^(1/2))) (логарифм от (гиперболический тангенс от ((x) в степени (1 делить на 2)))) - функции. Найдём значение производной функции в точке. [Есть ОТВЕТ!] log(atanh((x)^(1/2)))](/media/krcore-image-pods/176/hash/derivative/e/05/0f0242af7312ae8a12f0ffcbdd32f.png)
 Производная atan(sqrt(x))-1
Производная atan(sqrt(x))-1
 Производная (8/x+x^2)*sqrt(x)
Производная (8/x+x^2)*sqrt(x)